Two Theorems on Triangles and the Midpoints of Sides

There are two theorems in Euclidean geometry that describe the relationships between the lines drawn from the midpoints of the sides of a triangle and the other sides of the triangle.

.

Line Joining Two Midpoints Theorem

The line which joins the midpoints of two sides of a triangle is parallel
to the third side and equal to one-half of it.

Given: ABC is a triangle in which D and E are the midpoints of
sides AB and AC respectively.

Req'd to Prove: DE//BC and DE = ½ BC.

Proof: Produce DE to F making EF = DE. Join FC.

In triangles ADE and CEF:
AE = EC and DE = EF (given, proof),
Angle AED = ANGLE CEF (opposite angles)

(SAS)

so AD = FC (def. of congruence)
and DB = FC (axiom about equality)
since Angle ADE = Angle EFC (property of congruence)
AB//FC (alternate angles are equal)
So DBCF is a parallelogram (pair of opposite sides are equal and parallel)
therefore DE//BC and DF = BC (parallelogram)
but, DE = ½ DF (construction)
therefore DE = ½ BC

.

Line from Midpoint Parallel to Another Side Theorem

The line drawn from the midpoint of one side of a triangle
parallel to another side, bisects the third side.

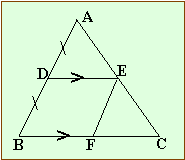
Given: D is the midpoint of side AB of triangle ABC.
DE is drawn parallel to BC and meets AC at E.

Req'd to Prove: E is the midpoint of AC (ie: AE = EC).

Proof: Draw EF // AB meeting BC at F.

Since DE // BC and EF // AB, DEFB is a parallelogram. (definition)
So EF = DB = AD (parallelogram thm.)
Now, in triangles ADE and EFC:
Angle DAE =Angle FEC and Angle AED =Angle ECF (corresponding angles)
AD = EF (proof)

(Angle, Side, Angle)
so AE = EC and E is the midpoint of AC. (congruence)

.

Example 1

Prove that the lines which join the midpoints of the three sides of a triangle
divide the triangle into four congruent triangles.

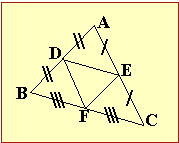
Given: In triangle ABC: D, E, and F are the midpoints of AB, AC and BC respectively.

Req'd to Prove: That DE, EF and DF divide triangle ABC into four congruent triangles.

Proof:Since D and E are mid points,

DE = ½ BC = BF = FC (midpoint thm., definition)
Similarly, DF = AE = EC and EF = AD = DB

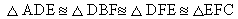So, (Side, Side, Side).

.

.

Example 2

Prove that any line drawn from the vertex of a triangle to the opposite side
is bisected by the line joining the midpoints of the other two sides.

Given: In triangle ABC, D and E are the midpoints of AB and AC respectively.

Req'd to Prove: DE bisects AF, any line from A to BC.

Proof: Draw AF, any line from A to BC meeting BC at F and DE at G.

DE//BC (midpoint thm.)
In triangle ABF, D is the midpoint of AB (given)
DG//BF (proof)
therefore G is the mid point of AF (midpoint thm.)
So DE bisects AF. (definition of a bisector)

.

This example illustrates a corollary (a theorem or proposition which follows readily from another theorem or proposition) of the midpoint theorems, which states that the line from the midpoint of one side of a triangle parallel to the base, bisects the altitude of the triangle.

Since the line joining the midpoints of two sides of a triangle is parallel to the third side, we can state that the line joining the midpoints of two sides of a triangle bisects the altitude of the triangle drawn to the third side.

Practice

Do the following questions in a  Given, Req'd to Prove, Proof format.
Be sure to draw a diagram and state the authority for the statements in the Proof.

1) Prove that the middle point of the hypotenuse of a right-angled triangle
is equidistant from the three vertices.

2) Through a point D in side AB of triangle ABC, a line is drawn parallel to BC meeting
AC in E. AC is produced to F so that CF = CE. Prove that DF is bisected by BC at G.

.

.

Solutions

1) Prove that the middle point of the hypotenuse of a right-angled triangle
is equidistant from the three vertices.

Given: In Triangle ABC, Angle B = 90^° and D is the midpoint of AC.

Req'd to Prove: AD = BD = CD.

Proof: Draw DE // BC meeting AB at E.

Angle AED = 90° (corresponding Angles)
AE = EB (midpoint theorem)
In triangles AED and BED:
AE = EB, Angle AED = 90° = Angle BED (proof)
DE = DE (common side)
So Triangle AED Triangle BED (Side, Angle, Side)
Therefore AD = BD (congruence)
but, AD = DC (given)
Therefore AD = BD = DC (axiom)

.

2) Through a point D in the side AB of Triangle ABC, a line is drawn parallel to BC and meets AC in E. AC is produced to F, making CF = CE. Prove that DF is bisected by BC.

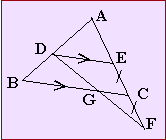
Given: D is any point in side AB of Triangle ABC. DE //BC and
intersects AC at E. AC is produced to F such that CF = CE.

Req'd to Prove: DF is bisected by BC. (DG = GF)

Proof: Join DF to intersect BC at G.

In Triangle DEF: C is the midpoint of EF (given)
CG // DE (given)
therefore G is the midpoint of DF (midpoint thm.)
therefore BC bisects DF

.

( Plane Geometry MathRoom Index )

(all content © MathRoom Learning Service; 2004 - ).