| Circles: Definitions and Metric Theorems |
Years ago, when I wanted to make a circular flower bed around the birch tree growing in my front yard, I attached a rope to the handle of my spade and then tied the other end of the rope around the tree leaving exactly the desired radius length of rope between the spade handle and the tree. I then walked around the tree planting the spade into the earth to make the outline of the flower bed's circumference. This activity was based on the definition of a circle.
| A circle is a path (locus) of points which are equidistant from a center point. The radius of the circle is the distance |
So, in my project, the tree acted as the center of the circle and the length of rope between the tree and the spade handle was the radius of my circular flower bed.
| intro | definitions | images | theorems | practice and solutions |
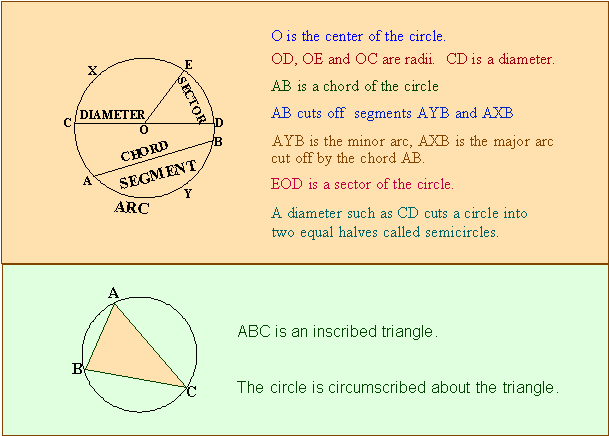
Circle Definitions
Chord: The line segment joining two points on the circumference of a circle.
Diameter: The longest chord. It passes through the center and equals 2 times the radius.
Segment: The two parts into which a chord divides a circle are called segments.
A segment is named with three letters: one at each end of the chord and another on the circumference between the initial two (as shown in the diagram).
Arc: The part of the circumference cut off by the end points of a chord.
Each chord creates two arcs: a major arc -- the larger of the two, and
a minor arc -- the smaller of the two arcs defined by the chord.
An arc is named with three points just like a segment.
Sector: A sector of a circle is the part of the circle bounded by two radii
and the arc intercepted between them.
Inscribed: If the vertices of a figure all lie on the circumference of a circle,
the figure is said to be inscribed in the circle.
Circumscribed: The circle around an inscribed figure is circumscribed around the figure.
| intro | definitions | images | theorems | practice and solutions |
.

.
| intro | definitions | images | theorems | practice and solutions |
.
Theorems on Chords in Circles
A/ Chord Right Bisector Theorems
 .
.
B/ Equal Chord / Arcs Theorems

definition-1:
a tangent is a line drawn from an external point that contacts the circle in exactly one point.
definition-2:
a secant is a line drawn from an external point that contacts the circle in two points.

.
C/ Constant Product Theorems
There are 3 Constant Product theorems that apply to the measures of
chords, secants and tangents.
Chord Thm: If 2 chords intersect, the products of their respective segments are equal.
Tangent/Secant Thm: For a tangent and secant drawn from an external point,
the square on the tangent equals the product of the secant and its external part.
Two Secant Thm: For 2 secants drawn from an external point, the respective
products of the secant and its external segment are equal.
.

.
Now use these theorems to answer these questions.
.
| intro | definitions | images | theorems | practice and solutions |
.
1/ Find the missing measures indicated by x, y, and/or z.
all measures are in cm.

.
Solutions
1/ a) We extend PO to meet the circle again at Q.
OQ = 9, so 3(21) = x 2 = y 2 = 64 so x = y = 8 cm.
b) By the Secant/Secant thm: x(2x) = 8(25) so x = 10 cm.
c) x = 2 cm. so x(6) = 3y so y = 4 cm.
Now, by the Tangent/Secant thm: z 2 = 5(5 + 3 + 4 ) so z = 7.75 cm.
.
| intro | definitions | images | theorems | practice and solutions |
.
( Plane Geometry MathRoom Index )
(all content © MathRoom Learning Service; 2004 - ).