
| DECIMALS |
What are Decimals?
Decimals are well behaved fractions because every one of them has a denominator that is a power of 10. This is why we call them well behaved. Our "whole number" system is based on place value and powers of 10, so decimals are user friendly fractions that we can write as whole numbers, placed to the right of or after the decimal point.Our money is based on a decimal system. There are one hundred cents in a dollar -- and when we write a dollar, we see $1.00. It sure looks like one hundred to me.
Fractions with Decimal Denominators
Any fraction with a denominator of 10, 100 or 1000 -- any power of 10 -- can be written as a decimal easily. We put the numerator of the fraction in the correct decimal place and it's done.
Examples:

Notice where we put the 7. In the first fraction -- seven tenths -- the denominator (10) has 1 zero in it, so we put the 7 in 1st place after the decimal. 100 has 2 zeros in it, so the 7 goes in the 2nd place after the decimal and so on.
WE MUST BE CAREFUL!! The same is not true on the left of the decimal point, because the first column is for the 1's. This often causes confusion because, on the fraction side of the decimal, there is no 1's column. The number of zeros in the denominator of the fraction tells us in what place to put the numerator. On the left (whole-number side) of the decimal, the number of digits we write tells us the place value. On the whole-number side of the decimal, hundreds are in the 3rd column -- not the second. That's for the 10's.
.
Decimal Place Value
Let's study the value of adjacent or neighboring columns whole numbers and decimals.
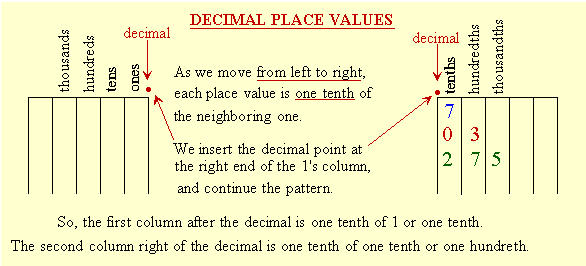
Notice the " TH " at the end of each decimal place name.
Like in one-fifth, 3-sevenths or 7-ninths, it indicates a fractional part of the whole.
7 in the tenths column, write 0.7 =  ; read seven-tenths.
; read seven-tenths.
0 in the tenths column, 3 in the hundredths write 0.03 =  ; read three-hudredths.
; read three-hudredths.
275 after the decimal, write 0.275 =  ; read 275-thousandths.
; read 275-thousandths.
Notice the 0 to the left of the decimal point in numbers that are smaller than 1.
It indicates or "holds" the 1's place or column. We always do this, because the decimal point is small, it looks like a period and so could be missed if we wrote .275 instead of 0.275. With a mixed number like 12.642, the decimal point is obvious.
Note: Many European countries (especially France and Belgium) use the comma ( , ) rather than the point ( . ) to indicate the decimal. So, if we write 3, 507 to mean three thousand five hundred and seven, someone from France might think it means 3.507. To avoid confusion, those of us who use the decimal point no longer use the comma to mark off groups of 3 digits. We now use a space where the comma would be like this: 19 201.3 instead of 19, 201.3
Changing the Denominator to a Power of 10
Since the denominator of any decimal's fraction equivalent is a power of 10, when we have to find a fraction equivalent for a decimal, and it's easy to make the denominator a power of 10 through multiplication by a fraction equal to 1, we can proceed like this.

We know that 5 × 20 = 100 so we multiplied seven-twentieths by 5/5 which equals 1 -- therefore we didn't change the value of our fraction -- we just wrote an equivalent fraction with a denominator of 100.
In the second case, we knew that 8 × 125 = 1000, so we multiplied by 8/8.
Rounding and Estimating Decimals
Now that we have a decimal system based on powers of 10 to express fractions, we can use the same approach to rounding and estimating that we do for whole numbers. First, we decide the place value to which we want to round, then we use the "halfway" rule that says if a number is exactly at or bigger than halfway to the next place or column, we round up. If the number is smaller than halfway to the next place or column, we round down. Let's look at some number lines and some rounded decimal numbers.

.
Adding and Subtracting Decimal Numbers
We add and subtract decimal numbers exactly the same way we add and subtract whole numbers. We carry powers of 10 to the next column left when we add, and we borrow from the next column left when we subtract. The trick is to line the decimals up so that we're adding and subtracting according to their place values. Another good trick is to put a zero (0) at the right end of the numbers when they don't end in the same decimal column.
Say we have to add 6.32 to 9.715. The 6.32 uses only 2 decimal places, while the 9.715 uses 3 decimal places. So to add them we write 6.320 not 6.32. We added nothing, (no thousandths) so we didn't change the value of the number, just its form. Now we can line it up with 9.715.
Examples:
Put zeros in these decimal numbers where needed, then add or subtract them.
Carry or borrow as always. Show all your work.
| 12.035 + 2.6
|
97.804 - 5.21
|
0.0014 + 2.679
|
14.338 - 8.4731
|
.
Now get a pencil, an eraser and a note book, copy the questions,
do the practice exercise(s), then check your work with the solutions.
If you get stuck, review the examples in the lesson, then try again.
![]()
Practice Exercises
1) Change the denominator to a power of 10, then write the decimal equivalent.
a)  |
b)  |
c)  |
d)  |
e)  |
2) Write equal to ( = ), greater than ( > ) or less than ( < ) between these numbers:
| a) 6.03 and 6.30 | b) $1.78 and $1.69 | c) 0.017 and 0.01700 | d) 256.33 and 256.29 |
3) Write these decimal numbers in order smallest to biggest:
| a) 2.7, 2.17, 2.07 | b) 1.006, 1.3, 1.015 | c) 8.63, 8.631, 8.59 | d) 4.026, 4.31, 4.035 |
4) Put zeros in these decimal numbers where needed, then add or subtract them.
| a) 12.803 - 10.92 | b) 54.9 + 7.318 | c) 0.674 - 0.0965 | d) 0.765 + 2.2867 |
.
![]()
Solutions
1) Change each denominator to a power of 10, then write the decimal equivalent.
a)  |
b)  |
c)  |
d)  |
e)  |
2) Write equal to ( = ), greater than ( > ) or less than ( < ) between these numbers:
| a) 6.03 < 6.30 | b) $1.78 > $1.69 | c) 0.017 = 0.01700 | d) 256.33 > 256.29 |
3) Write these decimal numbers in order smallest to biggest:
| a) 2.07 < 2.17 < 2.7 | b) 1.006 < 1.015 < 1.3 | c) 8.59 < 8.63 < 8.631 | d) 4.026 < 4.035 < 4.31 |
4) Put zeros in these decimal numbers where needed, then add or subtract them.
| 12.803 - 10.92
|
54.9 + 7.318
|
0.674 - 0.0965
|
0.765 + 2.2867
|
![]()