| PERIMETER AND AREA BASICS |
Perimeter: Getting Around the Outside
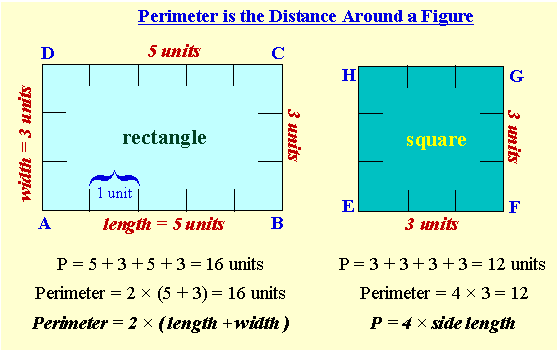
The Perimeter of a figure is the distance or measure around the outside.

length and width are sometimes called base and height.
To find the perimeter of a rectangle or square, we could add all the sides, but we could also take a shortcut since we know that pairs of sides in the rectangle and all sides in the square are equal.
A rectangle's perimeter (P) equals the sum of the length (l) and the width (w) times 2.
P = 2 × length + 2 × width = 2 × ( length + width ) = 2 × ( l + w )
For a square, the perimeter (P) equals 4 times the length of any side (s).
P = 4 × side length = 4s
For an irregular figure, Perimeter = the sum of the lengths of all the sides.
Examples:
1) The Perimeter of:
a) a parallelogram (//gm) with 2 sides = 7 in and 2 sides = 9 in. is 2 (7 + 9) = 32 inches.
b) a rectangle with length (l) = 14 ft. and width (w) = 10 ft. is 2 (14 + 10) = 2 (24) = 48 feet.
c) A Square with side (s) = 12 yards is 4 (12) = 48 yards.
![]()
.
Area: Covering Up the Inside
The things we use -- like paint, wallpaper and tiles -- to cover surfaces like walls and floors are always measured and priced in square units. The information on every paint can label includes the number of square feet or square meters of space, the paint will cover.
The measure of the surface covered by a closed figure is called AREA.
We measure area in SQUARE UNITS -- square inches, square feet, square miles --
because, to measure the area of a figure, we "square it off" and then count the squares.
We indicate or write square units like this: units², inches², ft².
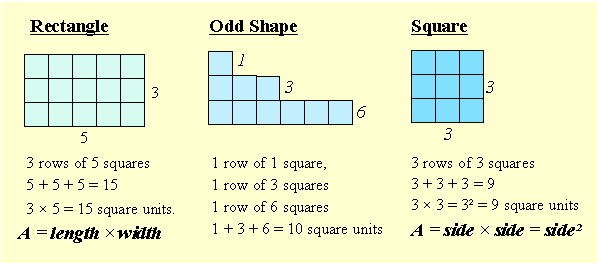
There's a shortcut for finding the area of a rectangle or a square.
For a rectangle the Area = length times width.
A = l × w
For a square, Area = the square of the side length.
A = s × s = s²
For an irregular figure, we count the squares.
.
Now get a pencil, an eraser and a note book, copy the questions,
do the practice exercise(s), then check your work with the solutions.
If you get stuck, review the examples in the lesson, then try again.
![]()
Practice Exercises
1) Find the perimeter of the figures below. Use the shortcut formula when you can.

2) Find the area of the figures above. Use the shortcut formula when you can.

3) Maria and her mother are making a tablecloth for a rectangular table that is 3 feet long
and 2 feet wide. They want the cloth to hang over the edge of the table on all sides by 1 foot.
a) What is the area of the tabletop?
b) What are the length and width of the tablecloth? (hint: draw the cloth as a rectangle)
c) What is the area of the tablecloth?
d) What is the difference between the area of the table and tablecloth?
e) What length of lace should Maria's mom buy to sew a lace trim all around
the outside edge of the cloth?
![]()
Solutions
1) a) A rectangle that is 4 units by 5 units. P = 2 (4 + 5) = 18 units.
b) A square that is 3 units per side. P = 4 × 3 = 12 units.
c) irregular shape, count steps around the outside = 22 units.
d) irregular shape, count steps around the outside = 21 units. (2 half steps make a whole on top)
2) a) A rectangle that is 4 units by 5 units. A = 4 × 5 = 20 square units.
b) A square that is 3 units per side. A = 3 × 3 = 3² = 9 square units.
c) irregular shape, count squares: A = 12 square units.
d) irregular shape, count squares: A = 13 square units.
3)

a) What is the area of the tabletop? 6 square feet
b) What are the length and width of the tablecloth? add 2 feet to each side: 5 ft. by 4 ft.
c) What is the area of the tablecloth? 20 square feet
d) The difference in the areas = 20 square feet - 6 square feet = 14 square feet.
e) length of lace she should buy = perimeter of the cloth = 2 (4 + 5) = 18 feet of lace.
![]()