| Angles in the Cartesian Plane |
Intro: Angles in Standard Position
Now that we know how to deal with the trig functions of angles, let's apply our knowledge to angles in the Cartesian Plane.
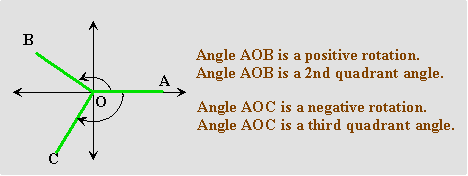
definition: An angle is in standard position if the vertex is at the origin and the initial side is on the positive x-axis.
The angle is formed by rotating the intial side about the origin in a positive or negative direction.
If the terminal side is in the 2nd quadrant, we call the angle a 2nd quadrant angle.
The same is true for the other quadrants.
If the terminal side is on an axis, the angle is called a quadrantal angle.
definition: positive rotation is counterclockwise.
definition: negative rotation is clockwise.
Both angles AOB and AOC are in standard position.
definition: coterminal angles have the same initial and terminal sides.
So, 30° and 390° are coterminal angles.
So are 60° and – 300° since they start and end at the same point.
When we know a point on the terminal side of an angle in standard position, we can find all its trig functions by constructing a right triangle with base on the x-axis. The coordinates of the terminal point tell us the lengths of the sides of the triangle. The hypotenuse is always positive whereas the sides can be either positive or negative depending on the quadrant.
When we build this right triangle, its base must be on the x-axis.
Example 1:
Find all 6 trig function values for angle AOB if it is in standard position with
point P(– 3, – 4) on its terminal side.
Solution:
We know the base and height of triangle OPC from the coordinates of P, therefore, we know the length of OP -- the hypotenuse.(Pythagoras)
Our triangle is the famous 3, 4, 5 right triangle so:
So now some of you are thinking that we found trig function values for angle POC rather than angle AOB. We did! The angles are coterminal and since trig function values are constants, we also found the values for angle AOB.
Sometimes, instead of knowing the coordinates of a point on the terminal side of the angle in question, we're told one of the trig function values and in which quadrant the angle lies. Since the given trig function tells us the lengths of 2 sides, we can figure out the length of the 3rd side of the triangle formed with the x-axis using Pythagoras.
Then we can list the required function values.
Example 2: Find the value of Cos A and Tan A given that Sin A = – 5/7 and A is in QIII.
Solution:
Since 
, we know the side opposite angle A measures 5 units and the hypotenuse = 7. The negative sign is because the point is in QIII.
So, the 3rd side (adjacent) of the triangle measures 
This is the x value -- and since we're in the 3rd quadrant, it must be negative.
Therefore 
, and 
.
If we don't know in what quadrant the angle lies, we would get 2 answers for both Cos A
and Tan A since the angle could be in 2 quadrants.
.
Example 3: Find Sin B and Cos B if Tan B = 6/7.
Solution: Since Tan B = 6/7, we know that the opposite side measures 6 units and the adjacent side measures 7 units. This means that the angle could be in the 1st or the 3rd quadrants, since, in the 3rd quad, Tan B is – 6/–7 = 6/7.
The hypotenuse of this triangle measures 
.
Therefore 
, and 
.
.
Now get a pencil, an eraser and a note book, copy the questions,
do the practice exercise(s), then check your work with the solutions.
If you get stuck, review the examples in the lesson, then try again.
Practice
Make diagrams for #1.
1) Find the 5 remaining trig functions of angle x given that:
| a) sin x = – 3/4, x in Q III | b) tan x = – 3/2, x in Q II |
| c) cos x = 12/13, x in Q IV | d) csc x = 5/3, tan x > 0 |
| e) sec x = – 7/4, cot x < 0 | f) cot x = 1, sin x > 0 |
Note: (for parts d, e and f, state in which quadrant the angle is found).
.
2)Match the angle in the left column with its coterminal angle in the right column.
| 1) – 390° | a) 77 ° |
| 2) 12° | b) 330° |
| 3) – 283° | c) – 348° |
.
1) Find the 5 remaining trig functions of x given that:
| a) sin x = – 3/4, x in Q III cos x = |
b) tan x = – 3/2, x in Q II sin x = |
| c) cos x = 12/13, x in Q IV sin x = – 5/13, tan x = – 5/12 |
d) csc x = 5/3, tan x > 0 sin x = 3/5, cos x = 4/5, tan x = 3/4, x in QI |
| e) sec x = – 7/4, cot x < 0 cos x = – 4/7, sin x = x in QII |
f) cot x = 1, sin x > 0 tan x = 1, cos x = sin x = x in QI |
.
2)Match the angle in the left column with its coterminal angle in the right column.
(answers are color-coded)
| 1) – 390° | a) 77° |
| 2) 12° | b) 330° |
| 3) – 283° | c) – 348° |
.
(all content of the MathRoom Lessons © Tammy the Tutor; 2004 - ).
, tan x = 
, cos x = 
, tan x = 
